1. Sic Bo Results Ufc
2. Sic Bo Results Football

• Live Sic Bo is a live dealer offering of the dice game that has been growing in popularity over the years. Live Sic Bo is going to be one of the more difficult live dealer games to find though, so don’t expect to see it everywhere.
• Sic Bo Try Sic Bo online for free in demo mode with no download or no registration required. Return to player. It will help us show others more accurate results.
• Sic bo is strictly a game of chance because every roll on the dice results a win or loss on any bet. In craps, some bets require certain rolls before they can become winning or losing bets, thus encouraging strategy.
• Sic Bo uses three dice to produce results. Players bet on a table layout full of betting options. Once bets are made, the dealer places the 3 dice in the roll box, gives them a good shake, and rolls the dice on the table.

The Mathematics of Sic Bo

Sic bo is one of two casino games involving dice, the other being craps. Sic bo is strictly a game of chance because every roll on the dice results a win or loss on any bet. In craps, some bets require certain rolls before they can become winning or losing bets, thus encouraging strategy.

By

Michael Shackleford

January 21, 2005

Sic Bo, meaning 'dice pair' is an ancient Chinese gambling game. Today it is one of the lesser known casino games and is often confined to designated rooms for Asian games. The game uses three dice and a table with a variety of betting options on the roll of those dice. The odds and table layout may also vary from place to place. If you must play Sic Bo I would suggest sticking to only the 'low' and 'high' bets.

Sic Bo Results Ufc

Following is a list of the bets available. The payoffs listed are for Atlantic City and the Mirage in Las Vegas. Other casino’s odds will vary.

• Small: Wins on total of 4-10, except for a three of a kind. Pays 1 to 1.
• Big: Wins on total of 11-17, except for a three of a kind. Pays 1 to 1.
• 4: Wins on total of 4. Pays 60 to 1.
• 5: Wins on total of 5. Pays 30 to 1.
• 6: Wins on total of 6. Pays 17 to 1.
• 7: Wins on total of 7. Pays 12 to 1.
• 8: Wins on total of 8. Pays 8 to 1.
• 9: Wins on total of 9. Pays 6 to 1.
• 10: Wins on total of 10. Pays 6 to 1.
• 11: Wins on total of 11. Pays 6 to 1.
• 12: Wins on total of 12. Pays 6 to 1.
• 13: Wins on total of 13. Pays 8 to 1.
• 14: Wins on total of 14. Pays 12 to 1.
• 15: Wins on total of 15. Pays 17 to 1.
• 16: Wins on total of 16. Pays 30 to 1.
• 17: Wins on total of 17. Pays 60 to 1.

• Two of a kind: Player may bet on any of the 15 possible two dice combinations (for example a 1 and 2). Bet wins if both numbers appear. Probability of winning is 13.89%. Pays 5 to 1.
• Double: Player may bet on any specific number (for example a 1). Player wins if at least 2 of the 3 dice land on that number. Probability of winning is 7.41%. Pays 10 to 1.
• Triple: Player may bet on any specific number (for example a 1). Player wins if all 3 dice land on that number. Probability of winning is 0.46%. Pays 180 to 1.
• Any Triple: Wins on any three of a kind. Pays 30 to 1.
• Individual Number: Player may bet on any specific number from 1 to 6. If chosen number appears 1 time bet pays 1 to 1, if it appears 2 times bet pays 2 to 1, and if it appears 3 times it pays 3 to 1.

The critical step in calculating the odds in Sic Bo is to find the probability of any given total in the throw of three dice. Following is a formula for s spots over n dice, taken from The Theory of Gambling and Statistical Logic by Richard A. Epstein, formula 5-14.

For example, let's look at the number of ways to get 11 spots over 3 dice.

int[(s-n)/6] = int[(11-3)/6] = int[1.33] = 1

The total would be 6^-3 * [-1⁰*combin(3,0)*combin(11-6*0-1,3-1) + -1¹*combin(3,1)*combin(11-6*1-1,3-1) ] =
1/218 * [1*1*combin(10,2) + -1*3*combin(4,2)] =
1/218 * [1*1*45 + -1*3*6] =
1/218 * [45-18] = 27/216 = 12.50%

Sic Bo Results Football

Alternatively, if you can program a computer that would probably be the fastest way to get the results.

Here is a simple function in C++.

Following is the output of the function.

If you don’t know how to program you’re going to have to do this the hard way. What I recommend is list every combination of 3 dice. To avoid the list being 6³=216 items long do not repeat the same combinations in different orders. In the interests of not listing the same number twice always order each combination from lowest to highest, not forgetting combinations with a pair or three of a kind.

So we start with 1,1,1.

Next would be 1,1,2.

Then 1,1,3; 1,1,4; 1,1,5; and 1,1,6.

Obviously you can’t roll a 7 with one dice so next we increment the second die.

1,2,?

The third die must be greater or equal to the second die so the next combination in full would be 1,2,2.

Next comes 1,2,3; 1,2,4; 1,2,5; and 1,2,6.

Then comes 1,3,3.

I hope you see the pattern. The whole list would look like the following.

Next we have to determine the number of permutations of each combination. A combination is a set without regard to order and a permutation is a set with regard to order.

With a three of a kind there is only one way permutation. For example if the three dice are 1,1,1 there is only one way to roll that a 1 each time.

If the combination is 1,1,2 there are three ways to roll that: 1,1,2; 1,2,1; and 2,1,1.

If all three dice are 1,2,3 there are six possible permutations: 1,2,3; 1,3,2; 2,1,3; 2,3,1; 3,1,2; 3,2,1

The general formula is that if you have a total of d dice and the totals of each number are x₁, x₂, x₃…x_n then the number of permutations are d!/(x₁!*x₂!*x₃*…*x_n). So the number of ways to get a three of a kind would be 3!/3! = 6/6 = 1. The number of ways to get a pair would be 3!/(2!*1!) = 6/(2*1) = 3. The number of ways to get three different numbers would be 3!/(1!*1!*1!) = 6/(1*1*1) = 6.

Next we go through the tedious process of adding the number of permutations for each total. For example a total of 6 has the following combinations with the corresponding number of permutations.

The final table will look like this, not unlike the result of the computer function earlier.

So now lets add a column to our list for the number of combinations of each set. Let’s also add a total for the three dice.

Now we can divide each total number permutations by the total number 3-dice permutations (216) to get the probability of each total.

Finally, we are ready to evaluate the expected value of each bet. The expected value is the ratio of the amount the player can expect to win to the amount he bets on any given bet. So a fair bet would an expected value of zero. A positive expected value would mean the player has the advantage. A negative expected value would mean the dealer has the advantage.

Let’s start with the 4 bet. This wins with a total of 4 and pays 60 to 1. For those who don’t know, '60 to 1' means if the player wins he wins 60 times his bet and KEEPS his original wager. Had the odds paid '60 for 1' the player would NOT keep his original bet. Most table games pay on a 'to 1' basis.

The probability of a total of 4 is 3/216 = 0.013889. Thus the probability of losing is 1-(3/216) = 1-0.013889 = 0.986111.

The expected value of any bet with only two possibilities, winning or losing, is:

(Probability of winning)*(Amount of win) + (Probability of losing)*(Amount of loss).

For the 4 bet the expected value is

0.013889 * 60 - 0.986111*-1 = -0.15278.

So, this tells us that for every dollar the player bets on a total of 4 he can expect to lose 15.278 cents on average. Or, the house edge is 15.278%.

The next table shows the expected value and how it was calculated for all bets of a total of 4 to 17.

Two of a kind

There are combin(6,2)=6!/(4!*2!)=15 ways to choose two numbers out of six. Each of these combinations is listed on the table and the player bet on as many as he wishes. If both numbers appear on the roll of the three dice then the player wins and is paid 15 to 1.

Let’s assume the player picks a 1 and 2 as his two numbers. What is the probability that both a 1 and 2 occur in the roll of 3 dice? One way to do this would be to note all the possible winning permutations:

Thus there are a total of 30 winning permutations.

There are 6³=216 total permutations, so the probability of winning is 30/216 = 1/36 = 0.1388889

The two of a kind bet pays 5 to 1. So the expected value is 0.1388889*5 + (1-0.1388889)*-1 = -0.16667. In other words the house edge is 16.67%.

Double

There are six double bets available, one for each number from 1 to 6. The player may be on any one or combination of bets. Any given bet wins if at least two of the three dice land on that number.

Let’s assume the player bets on the 1.

One way to solve it would be to note all the winning permutations:

Thus there are a total of 16 winning permutations.

There are 6³=216 total permutations, so the probability of winning is 16/216 = 0.0740741.

The double bet pays 10 to 1. So the expected value is 0.0740741*10 + (1-0.0740741)*-1 = -0.18518. In other words the house edge is 18.52% (ouch!).

Triple

Player may bet on any specific number (for example a 1). Player wins if all 3 dice land on that number.

There is obviously only one way to win this bet, so the probability of winning is 1/216 = 0.0046296. The bet pays 180 to 1 so the expected value is 0.0046296*180 + (1-0.0046296)*-1 = -0.16204. So the house edge is 16.204%.

Any Triple

The Any Triple bet pays if any three of a kind is thrown. There are obviously six winning combinations (1,1,1; 2,2,2; 3,3,3; etc.). So the probability of winning is 6/216 = 0.027778. The bet pays 30 to 1 so the expected value is 0.027778*30 + (1-0.027778)*-1 = -0.13889. So the house edge is 13.89%.

Low

The low bet wins if the total of the three dice is 3 to 10, without being a three of a kind. The probability of any total 10 or less is exactly 50%. The average number on any one die is (1+2+3+4+5+6)/6 = 21/6 = 3.5. So the average of three dice is 3*3.5 = 10.5. It stands to reason that the probability of getting under or over 10.5 is 50%.

However the bet loses on a three of a kind. There are 3 three of a kinds that would turn a winner into a loser: 1,1,1; 2,2,2; and 3,3,3. So the probability of having a total of 10 or less as a three of a kind is 3/216 = 0.0188889. So the overall probability of winning is 0.5 – 0.188889 = 0.4861111. The bet pays 1 to 1 so the expected value is 0.4861111*1 + (1-0.4861111)*-1 = -0.02778. Thus the house edge is 2.78%.

High

The high is just the opposite of the low bet, so it stands to reason the house edge would also be 2.78%.

Individual Number

Player may bet on any specific number from 1 to 6. If chosen number appears 1 time bet pays 1 to 1, if it appears 2 times bet pays 2 to 1, and if it appears 3 times it pays 3 to 1. Probability of 1 match is 34.72%, 2 matches is 6.94%, 3 matches is 0.46%.

Let’s assume the player picks the number one.

There is only one way to get three ones: 1,1,1. So the probability of three ones is 1/6³ = 1/216.

Following are the ways to get two 1’s and the number of permutations of each.

So the probability of two ones is 15/6³ = 15/216.

Following are the ways to get one 1 and the number of permutations of each.

So the probability of two ones is 75/6³ = 75/216.

Another way to arrive at the probability of one 1 would be find the probability that the first die is a one and the second and third are not:

Pr(one)*Pr(not one)*Pr(not one) = (1/6)*(5/6)*(5/6) = 25/216.

However the one could appear in the first, second, or third position, so multiply by 3: 3*(25/216) = 75/216.

The probability of rolling zero ones is Pr(not one)*Pr(not one)*Pr(not one) = (5/6)*(5/6)*(5/6) = (5/6)³ = 125/216.

The following return table shows the possible outcomes, and the number of combinations, probability, and return of each. The return is the product of the probability and the win or loss to the player.

So the total expected return is -0.0787, or the house edge is 7.87%.

Sic Bo section at the Wizard of Odds.
How to calcualte the 3-dice permutation in Visual Basic.

Sic bo, or “precious dice,” is a casino game that originated in Asia. In fact, it was exclusively played in Asia up until the early 1900s.

Chinese immigrants brought this game to the United States by the turn of the twentieth century. Even then, though, sic bo didn’t find its way into American gambling venues until much later.

Sic bo has only recently begun gaining traction in Europe as well. The UK finally legalized sic bo for casino play in 2002.

Given its relative newness outside of Asia, sic bo can feel like an intimidating game. Its board is a confusing mix of dominoes and dice combinations.

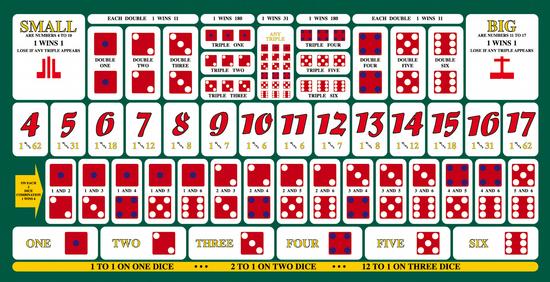
It also carries the reputation for having a really high house edge. Therefore, you may be turned off before you even get started with this game.

Nevertheless, sic bo is still a quality casino game that’s worth trying. You can read more about the reasons why you needn’t fear it below.

Despite its complicated board, sic bo isn’t very difficult to understand. The general premise is that you wager on the outcome of three dice. Assuming you’ve played craps before, you’ll be perfectly comfortable with this concept.

Each round begins with you placing your chips on the desired spot(s) on the board. Once you and all other gamblers have finished betting, the dealer will shake a small cage that contains three pair of dice.

After the dealer finishes shaking the dice, they open the cage and reveal the combination. If you win, your bet will be paid according to the pay table.

Fairly Low House Edges on Some Wagers

The tough part about playing sic bo for real money is avoiding the many sucker bets. The board contains many terrible wagers that feature house edges as high as 29.2%.

However, nobody’s forcing you to making any such bets. Instead, you can skip all the atrocious wagers and concentrate on “Big” and “Small.”

Both carry a 2.78% house edge, which is low in the grand scheme of casino gambling. For reference, European roulette, a well-liked game, carries a similar 2.70% house advantage.

Here’s more info on these wagers.

• Big – You win with a total combination of 11 through 17. You lose with anything under 11 and three of a kinds (4:4:4 and 5:5:5). Winning bets pay at 1 to 1.
• Small – You win with a total combination of 4 through 10. You lose with anything over 10 and on triples (2:2:2 and 3:3:3). Winning wagers pay at 1 to 1.

In contrast to casino games like blackjack and video poker, you don’t have to think too hard with this one. You achieve optimal strategy simply by betting on Big or Small.

You just need to find the spaces on the board that are designated by “Big” (upper right-hand corner) or “Small” (upper left-hand corner).

The other part of sic bo strategy is to avoid the other bets. The board is a minefield of awful prop bets with house edges ranging from 7.4% to 29.2%.

You might as well leave the casino and play the lottery — which pays just as poorly — if you’re thinking about other sic bo wagers.

Exciting Atmosphere

Nothing in the table games section beats craps with regard to atmosphere. However, sic bo does present a fun environment in its own right.

First off, this game is still fairly new in its modern casino format. Assuming you haven’t tried sic bo before, then you’ll get to experience something fresh.

Secondly, the sheer anticipation of waiting on the dealer to finish shaking up the dice and revealing the combination is also exciting.

Sure, you may not get to roll the dice like in craps. But you still experience the thrills of dice-based gambling.

Although sic bo is primarily considered an Asian game, it’s based on English dice-based games in Grand Hazzard and Chuck-a-luck.

The former, which is based on the even older game Hazard, is played with three dice just like sic bo. Grand Hazzard sees the three dice shaken in and rolled out of a cup.

Chuck-a-luck, or “sweat cloth,” was spun off Grand Hazzard. Its three dice are shaken and rolled out of a birdcage-like device.

Earlier, I described the dire situation you face when going away from the Big and Small bets. Long story short, you don’t want to make either of these wagers.

But sic bo isn’t any different from craps in this regard. There are also a lot of really bad craps wagers.

This game does offer a few solid wagers, though, in pass line (1.41% house edge), come (same), don’t pass line (1.36% house edge), and don’t come (same).

Assuming you venture too far away from these bets, then you can hit some very high house advantages. The worst of the bunch is Any 7 (single-roll 7), which carries a 16.67% casino edge.

If you’ve heard that sic bo is the worst game in the casino regarding house edge, then you can discount this thought. Craps also features some risky bets too.

Conclusion

Sic bo is by no means brand new to the casino landscape. However, it hasn’t had quite as much time to warm up gamblers as many other table games.

Therefore, sic bo is often stereotyped as an intimidating or even bad game. In reality, it’s not much worse than craps regarding these aspects.

First off, sic by has pretty simple game play. You mainly need to know what each betting square means. As long as you can figure out your desired wager, then you’ll have no trouble playing.

Once you place your bet(s), the dealer will shake up the dice and roll them. Your results depend upon the three-dice combination.

Unfortunately, most sic bo bets are really bad in terms of house edge. But Big and Small allow you to bring the house edge down to 2.78%. You should never go away from these wagers if you want the best odds of winning.

Sic bo also presents a fun-and-exciting atmosphere. Everything from waiting for the dice to be shaken to seeing the bones roll out of the cage is thrilling.

Assuming you’re interested in playing sic bo, then you can find it at both land-based casinos and most of the top online casinos. If you’re on the fence about playing it, then you can simply play free sic bo online.

Of course, half the excitement is enjoying this game in land-based casinos. That said, you haven’t truly experienced sic bo until you’ve tried it in a brick-and-mortar venue.